
by Niro Kasahara, MD; Mateus L. Matuoka, MD; Katia S. Santos, MD; Natasha F.S. Cruz, MD; Alvaro R. Martins, MD; and Stanley Nigro, MD
Drs. Kasahara, Matuoka, Santos, and Cruz are with the Department of Ophthalmology, Irmandade da Santa Casa de Misericordia de Sao Paulo in Sao Paulo, Brazil. Dr. Kasahara is also with Santa Casa de Sao Paulo School of Medical Sciences in Sao Paulo, Brazil. Drs. Martins and Nigro are with Clinical Pathology Laboratory, Irmandade da Santa Casa de Misericordia de Sao Paulo in Sao Paulo, Brazil, and Santa Casa de Sao Paulo School of Medical Sciences in Sao Paulo, Brazil
Funding: No funding was provided.
Disclosures: The authors have no conflicts of interest relevant to the content of this article.
Innov Clin Neurosci. 2018;15(11–12):27–29
Abstract: Objective: There has been an interest regarding the possible role intracranial pressure (ICP) plays in the pathogenesis of the glaucoma optic neuropathy. A major impediment to the understanding of the possible role of ICP on glaucoma is the reliance on alternative noninvasive methods to measure the ICP. A formula was developed to estimate the ICP for the Chinese population. This was cross-sectional, observational study aimed to compare the predicted ICP with the real ICP measured with an aneroid manometer through lumbar puncture in a cohort of 39 subjects. Main points of discussion: The Bland-Altman plot of the differences between the two techniques against their averages revealed that most data points were sited between the two limits of agreement. Conclusion: The results of this study favor the use of the equation as a proxy method to predict ICP and it could be used in clinical studies.
Keywords: Glaucoma, cerebrospinal fluid pressure, translaminar pressure, clinical study, clinical trial, trial methodology, measurement tool
Glaucoma optic neuropathy is characterized by a slow progressive degeneration of retinal ganglion cells and their axons, which ultimately leads to blindness if untreated. The biological basis of the disease is not yet fully understood, and the factors contributing to its progression include high intraocular pressure (IOP), family history, African ancestry, cardiovascular disease, migraine, and peripheral vasospasm.1 Recent studies have implicated the role of intracranial pressure (ICP) as a major contributor to glaucoma.2,3 The lamina cribrosa separates the intraocular space from the subarachnoid cerebrospinal fluid space. The orbital ICP and the optic nerve tissue pressure form the retro-laminar counter-pressure against the IOP and thus are part of the translamina cribrosa pressure difference and gradient. Assuming that an elevated translaminar pressure difference and a steeper translaminar pressure gradient are important for glaucomatous optic nerve damage, a low orbital ICP might therefore play a role in the pathogenesis of glaucoma.4,5
A major impediment to the expansion on the acumen of the possible role of ICP on glaucoma pathogenesis is the reliance on alternative noninvasive and relatively inexpensive methods to measure the ICP. Thus, there has been substantial interest in developing noninvasive techniques for assessment of ICP. Some approaches were reported, although due to inaccuracy, none seem to provide a complete solution.6 Using a multivariate analysis, Jonas et al7 developed a formula to estimate the ICP for the Chinese population. Since then, the equation has not been tested or validated in different populations. Hence, we undertook a cross-sectional, observational study to compare the predicted with the measured ICP and to validate the equation for clinical studies in a small validation cohort of Brazilian patients.
The Institution Ethics Committee approved the study. Patients scheduled to have diagnostic lumbar puncture were invited to participate. All participants signed informed consent after being fully aware of the procedures and potential risks.
After a brief medical interview, patients undertook the study procedures. Height (cm) was measured to the nearest 0.1cm with the patient’s back against the wall, without shoes, with feet together, using a wall-mounted stadiometer. Body weight (kg) was measured to the nearest 0.1kg on a calibrated manual platform scale with the patient wearing light clothing. Body mass index (BMI) was calculated as the body mass divided by the square of the body height (kg/m2) for each participant. Brachial arterial blood pressure was measured with the aneroid sphygmomanometer (Gurin Products, LLC, Tustin, California, USA) in the right arm with the patient in a sitting position.
The ICP was measured by lumbar puncture using aseptic technique.8 Each patient was positioned in the left lateral decubitus, with the vertebrae in line in the horizontal plane, the head in a neutral position, and the knees flexed. Once the skin was sterile, an intradermal bleb of 0.5mL of 1% lidocaine was administered to produce cutaneous anesthesia. The lumbar puncture needle (22G Whitacre spinal needle, BD Becton, Dickinson and Company, Franklin Lakes, New Jersey, USA) was inserted at an angle that allowed it to pass between the spinous processes of L3 and L4 having the Tuffier’s imaginary line drawn across the highest points of both the iliac crests as the anatomical landmark. The trajectory was adjusted, gradually proceeding until a “give” was felt on passing through the ligamentum flavum. Once the needle was sufficiently advanced, the stylet was slowly withdrawn to observe if cerebrospinal fluid (CSF) would emerge. If, after five seconds, it did not, the stylet was replaced and the needle advanced another 2 to 3mm and checked again for CSF. Once CSF was obtained, the Claude aneroid manometer (Spengler SAS, La Robôle, France) was connected and the ICP measured. Last, a small sterile dressing was placed on the puncture site.
After all data were collected, the predictive ICP was calculated according to the equation of Xie et al:9 ICP=(0.44×BMI)+(0.16×DBP)–(0.18×age)–1.91. The Bland-Altman analysis was done to compare predicted and measured ICP with 95-percent limits of agreement.
A small cohort of 39 subjects enrolled in the study. Demographic features of the sample are shown on Table 1. Figure 1 displays the Bland-Altman plot of the differences between the two techniques against their averages. Except for three patients—one with infectious meningitis, one with multiple sclerosis, and one intracranial hypertension—most data points were sited between the two limits of agreement (the differences within mean±1.96 standard deviation [SD] are not clinically important) and thus, the two methods were considered to be in agreement.

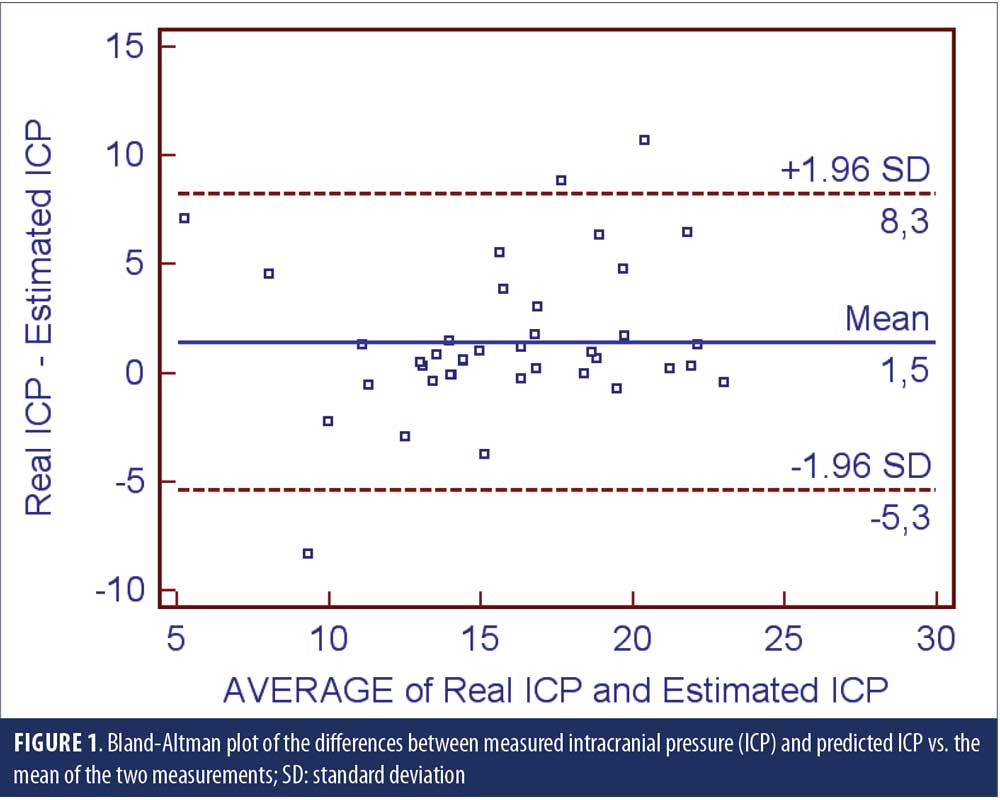
Xie et al9 created a formula to estimate the ICP from 72 patients who consecutively underwent lumbar puncture for diagnosis and treatment of suspected neurological diseases, such as peripheral neuropathy, intracranial hypertension, spontaneous intracranial hypotension, cavernous sinus syndrome, meningitis, multiple sclerosis, unilateral ischemic optic neuropathy, unilateral optic neuritis, optic nerve atrophy, and head injury. Likewise, the sample used in our study only comprised patients with a number of neurological conditions, due to the ethical barrier of performing lumbar punctures on normal subjects for study purposes. The authors randomly divided the group into a training group of 32 patients and a test group with the remaining 42 patients, with no significant differences between groups in age, sex, body height and weight, BMI, intraocular pressure, retinal nerve fiber layer thickness, and arterial blood pressure. Based on the multivariate analysis in the training group, ICP was best described by the formula as a function of BMI, diastolic arterial pressure, and age, concurring with previous investigations that had also reported on associations between higher ICP and younger age, higher BMI, and higher blood pressure.10,11 Applying the formula to the independent test group revealed that the measured and calculated ICP did not differ significantly (P=0.29); intra-class correlation and a Bland-Altman analysis revealed satisfying agreement as well.7 The authors, however, have not validated the formula using a more robust statistical approach using bootstrapping methods in an independent sample.12
Conclusion
Although initially developed in a Chinese population, the results of this study favor the use of the Jonas equation as a proxy method to predict ICP. We do not propose the use of the equation on clinical grounds (i.e., for diagnosis or monitoring of patients with neurological disorders); however, the equation might be a suitable surrogate method to predict ICP in large clinical studies.
References
- Weinreb RN, Khaw PT. Primary open-angle glaucoma. Lancet. 2004;363(9422):1711–1720.
- Berdahl JP, Allingham RR, Johnson DH. Cerebrospinal fluid pressure is decreased in primary open-angle glaucoma. Ophthalmol. 2008;115(5):763–768.
- Berdahl JP, Fautsch MP, Stinnett SS, Allingham RR. Intracranial pressure in primary open angle glaucoma, normal tension glaucoma, and ocular hypertension: a case-control study. Invest Ophthalmol Vis Sci. 2008;49(12):5412–5418.
- Jonas JB. Role of cerebrospinal fluid pressure in the pathogenesis of glaucoma. Acta Ophthalmol. 2011;89(6):505–514.
- Jonas JB, Wang N. Cerebrospinal fluid pressure and glaucoma. J Ophthalmic Vis Res. 2013;8(3):257–263.
- Kawoos U, McCarron RM, Auker CR, Chavko M. Advances in intracranial pressure monitoring and its significance in managing traumatic brain injury. Int J Mol Sci. 2015;16(12):28979–28997.
- Jonas JB, Wang N, Wang YX, et al. Body height, estimated cerebrospinal fluid pressure and open-angle glaucoma. The Beijing Eye Study 2011. PLoS One. 2014;9(1):e86678.
- Doherty CM, Forbes RB. Diagnostic lumbar puncture. Ulster Med J. 2014;83(2):93–102.
- Xie X, Zhang X, Fu J, et al. Intracranial pressure estimation by orbital subarachnoid space measurement. Crit Care. 2013;17(4):R162.
- Berdahl JP, Fleischman D, Zaydlarova J, et al. Body mass index has a linear relationship with cerebrospinal fluid pressure. Invest Ophthalmol Vis Sci. 2012;53(3):1422–1427.
- Ren R, Wang N, Zhang X, et al. Cerebrospinal fluid pressure correlated with body mass index. Graefes Arch Clin Exp Ophthalmol. 2012;250(3):445–446.
- Efron B. The bootstrap and modern statistics. J Am Stat Assoc. 2000;95(452):1293–1296.





